python 和c++实现旋转矩阵到欧拉角的变换方式
在摄影测量学科中,国际摄影测量遵循OPK系统,即是xyz转角系统,而工业中往往使用zyx转角系统。
旋转矩阵的意义:描述相对地面的旋转情况,yaw-pitch-roll对应zyx对应k,p,w
#include <iostream>
#include<stdlib.h>
#include<eigen3/Eigen/Core>
#include<eigen3/Eigen/Dense>
#include<stdlib.h>
using namespace std;
Eigen::Matrix3d rotationVectorToMatrix(Eigen::Vector3d theta)
{
Eigen::Matrix3d R_x=Eigen::AngleAxisd(theta(0),Eigen::Vector3d(1,0,0)).toRotationMatrix();
Eigen::Matrix3d R_y=Eigen::AngleAxisd(theta(1),Eigen::Vector3d(0,1,0)).toRotationMatrix();
Eigen::Matrix3d R_z=Eigen::AngleAxisd(theta(2),Eigen::Vector3d(0,0,1)).toRotationMatrix();
return R_z*R_y*R_x;
}
bool isRotationMatirx(Eigen::Matrix3d R)
{
int err=1e-6;//判断R是否奇异
Eigen::Matrix3d shouldIdenity;
shouldIdenity=R*R.transpose();
Eigen::Matrix3d I=Eigen::Matrix3d::Identity();
return (shouldIdenity-I).norm()<err?true:false;
}
int main(int argc, char *argv[])
{
Eigen::Matrix3d R;
Eigen::Vector3d theta(rand() % 360 - 180.0, rand() % 360 - 180.0, rand() % 360 - 180.0);
theta=theta*M_PI/180;
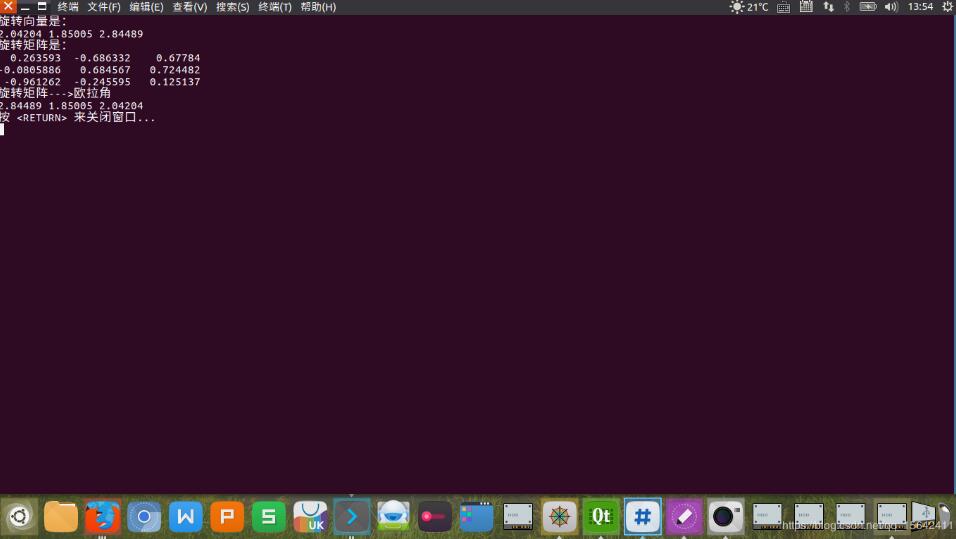
cout<<"旋转向量是:\n"<<theta.transpose()<<endl;
R=rotationVectorToMatrix(theta);
cout<<"旋转矩阵是:\n"<<R<<endl;
if(! isRotationMatirx(R)){
cout<<"旋转矩阵--->欧拉角\n"<<R.eulerAngles(2,1,0).transpose()<<endl;//z-y-x顺序,与theta顺序是x,y,z
}
else{
assert(isRotationMatirx(R));
}
return 0;
}
#!/usr/bin/env python3
# -*- coding: utf-8 -*-
import cv2
import numpy as np
import math
import random
def isRotationMatrix(R) :
Rt = np.transpose(R)
shouldBeIdentity = np.dot(Rt, R)
I = np.identity(3, dtype = R.dtype)
n = np.linalg.norm(I - shouldBeIdentity)
return n < 1e-6
def rotationMatrixToEulerAngles(R) :
assert(isRotationMatrix(R))
sy = math.sqrt(R[0,0] * R[0,0] + R[1,0] * R[1,0])
singular = sy < 1e-6
if not singular :
x = math.atan2(R[2,1] , R[2,2])
y = math.atan2(-R[2,0], sy)
z = math.atan2(R[1,0], R[0,0])
else :
x = math.atan2(-R[1,2], R[1,1])
y = math.atan2(-R[2,0], sy)
z = 0
return np.array([x, y, z])
def eulerAnglesToRotationMatrix(theta) :
R_x = np.array([[1, 0, 0 ],
[0, math.cos(theta[0]), -math.sin(theta[0]) ],
[0, math.sin(theta[0]), math.cos(theta[0]) ]
])
R_y = np.array([[math.cos(theta[1]), 0, math.sin(theta[1]) ],
[0, 1, 0 ],
[-math.sin(theta[1]), 0, math.cos(theta[1]) ]
])
R_z = np.array([[math.cos(theta[2]), -math.sin(theta[2]), 0],
[math.sin(theta[2]), math.cos(theta[2]), 0],
[0, 0, 1]
])
R = np.dot(R_z, np.dot( R_y, R_x ))
return R
if __name__ == '__main__' :
e = np.random.rand(3) * math.pi * 2 - math.pi
R = eulerAnglesToRotationMatrix(e)
e1 = rotationMatrixToEulerAngles(R)
R1 = eulerAnglesToRotationMatrix(e1)
print ("\nInput Euler angles :\n{0}".format(e))
print ("\nR :\n{0}".format(R))
print ("\nOutput Euler angles :\n{0}".format(e1))
print ("\nR1 :\n{0}".format(R1))
以上这篇python 和c++实现旋转矩阵到欧拉角的变换方式就是小编分享给大家的全部内容了,希望能给大家一个参考,也希望大家多多支持我们。
赞 (0)
